Equivalent Fractions

Pizza

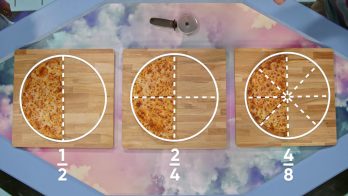
April, Adesina, and Marcos have leftover pizza. Everyone's pizza started out the same size and shape. April’s pizza was cut into 2 equal pieces, and she has one piece left. This is represented with [ggfrac]1/2[/ggfrac]. Adesina’s pizza was cut into 4 equal pieces and she has 2 pieces left. This is represented with [ggfrac]2/4[/ggfrac]. Marcos’ pizza was cut into 8 equal pieces and he has 4 pieces left. This is represented with [ggfrac]4/8[/ggfrac]. Who has more pizza left? Visually, it looks like all three have the same amount of pizza, but what’s the difference? Even though the fractions have different numerators and denominators, they represent the same amount of a whole pizza. They are called equivalent fractions. We prove this using a pizza cutter: if we double the number of slices by cutting April’s slice in half, we turn [ggfrac]1/2[/ggfrac] into [ggfrac]2/4[/ggfrac]. If we again double the number of slices by cutting each [ggfrac]1/4[/ggfrac] in half, we turn [ggfrac]2/4[/ggfrac] into [ggfrac]4/8[/ggfrac]. Now you try: Draw a pizza that has [ggfrac]2/3[/ggfrac] left. Draw lines to show that this is the same amount as [ggfrac]4/6[/ggfrac].

Dividing Clay

April and Marcos have a block of clay to use for an art project. April wants to use [ggfrac]1/2[/ggfrac] of the block, while Marcos wants to use [ggfrac]8/16[/ggfrac] of the block. How can we find out if these amounts are the same? We start by cutting the block into two halves. Now we can show what [ggfrac]1/2[/ggfrac] looks like. Next, cut each of the pieces in half to show what [ggfrac]2/4[/ggfrac] looks like. If we repeat this step, we can show what [ggfrac]4/8[/ggfrac] looks like, and finally, what [ggfrac]8/16[/ggfrac] looks like. We can represent all of these pieces using the same amount of clay, so [ggfrac]1/2[/ggfrac], [ggfrac]2/4[/ggfrac], [ggfrac]4/8[/ggfrac], and [ggfrac]8/16[/ggfrac] are all equivalent fractions. To make equivalent fractions using math, we multiply both the denominator and the numerator by 2 each time. For [ggfrac]1/2[/ggfrac], we multiply 1 × 2 = 2, and 2 × 2 = 4 to get [ggfrac]2/4[/ggfrac]. They are equivalent. Each time we double the numerator, we must double the denominator as well to get an equivalent fraction. In fact, we can find an equivalent fraction by multiplying the numerator and denominator by any number, as long as it is the same number. Now you try: Find 3 fractions that are equivalent to [ggfrac]1/3[/ggfrac].

Sandwich Deli

Adesina shows April and Marcos how comparing equivalent fractions helps us serve lunch. How can we check if [ggfrac]2/3[/ggfrac] is equal to [ggfrac]6/9[/ggfrac] or [ggfrac]10/15[/ggfrac]? Start with [ggfrac]2/3[/ggfrac]. If we multiply the numerator and denominator by 2, we get [ggfrac]4/6[/ggfrac], so it is an equivalent fraction. If we instead multiply the numerator and denominator by 3, we get [ggfrac]6/9[/ggfrac]. [ggfrac]6/9[/ggfrac] is an equivalent fraction. Multiplying by 4, we get [ggfrac]8/12[/ggfrac], and multiplying by 5, we get [ggfrac]10/15[/ggfrac]. All of these fractions are equivalent, and we can find an infinite number of equivalent fractions, multiplying both the numerator and denominator by the same number. Now you try: Is [ggfrac]2/5[/ggfrac] equivalent to [ggfrac]14/35[/ggfrac]? How do you know?

Chocolate Bars