Intro to Negative Numbers

Intro to negative numbers. A tank of liquid nitrogen is at –196°C. A package of dry ice is at –78°C. These are examples of negative numbers. A negative number has a value that is less than zero. On a horizontal number line, these numbers are to the left of zero. Counting negative numbers is a lot like counting positive numbers: negative 1, negative 2, negative 3, and so on. Try this one yourself: Count down from 5 to –5.

Temperatures can be negative numbers. When you look at weather forecasts, you can see negative numbers. Temperatures can contain a negative sign, such as –1°C, -196°C, and -15°C. You can count by counting down on a thermometer: 2°C, 1°C are positive numbers. Next is 0°C which is neither positive nor negative. Then –1°C, -2°C, -3°C. The numbers 1 and –1 are an example of opposite numbers, because they are the same distance from zero, in opposite directions. Try this one yourself: Explain why 8 and –8 are opposites. Use a real-world scenario and a number line to support your answer.

You can compare negative numbers. You can compare negative numbers by imagining where the numbers would be on a number line. Like with positive numbers, lesser numbers are on the left and greater numbers are on the right. In Yellowknife, Canada, the temperature today is –15°C. In Norilsk, Russian, it is –30°C. Which place is colder? Colder means lesser temperature, so you need to find which number is lesser. –30 is to the left of –15, so –30 < –15. Norilsk is colder than Yellowknife. Try this one yourself: Compare –45 and –16.

Your bank balance can also be negative. We can use negative numbers to express the amount of money owed. For example, Emily wants to buy a T-shirt online. She has $10 in her bank account. The T-shirt Emily wants costs $15. If she tries to buy that T-shirt, she doesn’t have enough money. Her bank allows the purchase to go through, but then Emily’s account balance is –$5. This means she has less than $0 in her bank account because she owes the bank $5. She has to give $5 back to get to a balance of $0 again. Try this one yourself: If Amari starts with $25 in his bank account, spends it all, and then buys something for $12, what is Amari’s account balance?

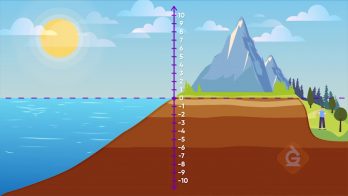
Your elevation can be negative. Another use of negative numbers is understanding elevation, the distance above or below sea level. Some areas are above sea level and are represented with positive numbers. Areas at sea level are represented with the number zero. Some areas are below sea level and are represented with negative numbers. The top of Mount Everest, a very large mountain, is 8,846 meters above sea level. On the other hand, the lowest elevation in the United States is in Death Valley, California, at 86 meters below sea level. This elevation can be represented by the number –86. –86 is 86 units below zero on a vertical number line. Try this one yourself: The Dead Sea in Israel is 430 meters below sea level. What number represents the elevation of the Dead Sea?

Use absolute value to compare liquid nitrogen and dry ice. A tank of liquid nitrogen is at –196°C. A package of dry ice is at -78°C. Both are very cold. How do they compare? –78°C > -196°C, because –78 is closer to zero than –196. –196 < –78, because –196 is further from zero than –78. These comparisons can look confusing because with positive numbers, we write 78 < 196 and 196 > 78. When comparing two negative numbers, the number with the greater absolute value is actually the lesser number. Absolute value is the distance of a number from 0 on a number line. For example, the absolute value of –196, also written as |–196|, is 196, since it is 196 units from 0. The absolute value of –78, also written as |–78|, is 78. Since –196 has the greater absolute value, it’s the lesser number. Try this one yourself: In Harbin, China, January temperatures are around –20℃. In International Falls, Minnesota, January temperatures are around –10℃. Which place is warmer? Use absolute value to explain your answer.