Read About Division with Unit Fractions & Whole Numbers

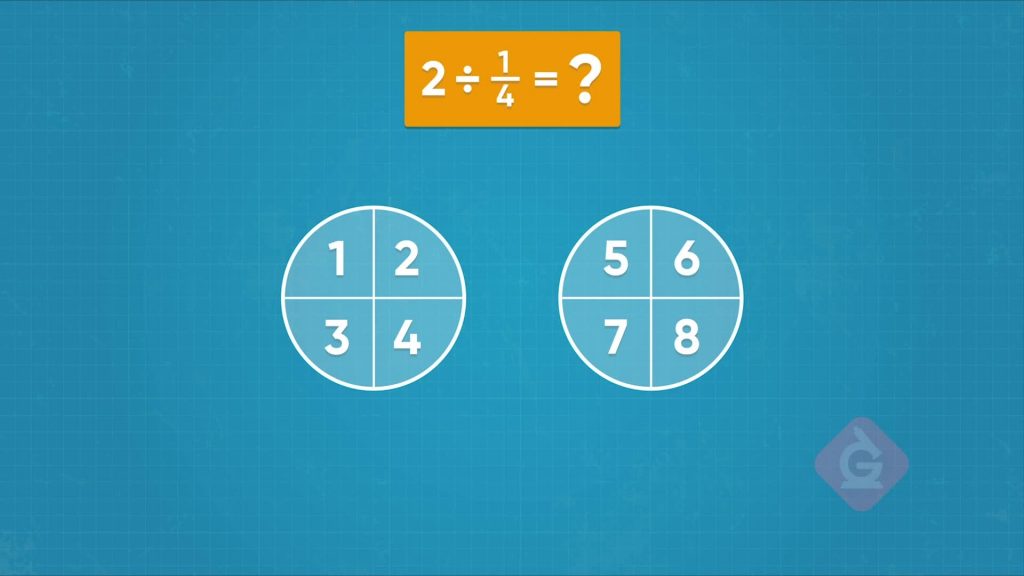
Slime Let’s say you want to make some slime. You need [ggfrac]1/4[/ggfrac] cup of glue for each batch. You have 2 cups of glue. You want to find out how many batches of slime you can make. You can write a division equation to show what you are trying to find: 2 ÷ [ggfrac]1/4[/ggfrac] = ? You can draw two circles to represent the 2 cups of glue. Then partition each circle into four equal parts. Each equal part represents [ggfrac]1/4[/ggfrac] of the circle, or [ggfrac]1/4[/ggfrac] cup. Count the number of one-fourths. Each fourth represents one batch of slime that you can make. You can make 8 batches of slime. Try this one yourself. You are mixing blue and yellow paint to create green paint. For each batch of paint, you need [ggfrac]1/3[/ggfrac] cup of yellow paint. You have 3 cups of yellow paint. How many batches of green paint can you make with that amount of yellow paint?

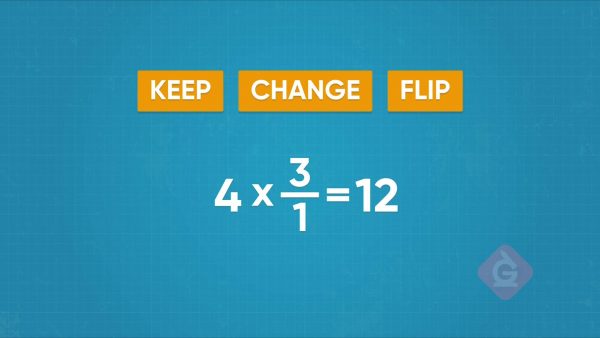
Guitar Strings Let’s say you have 4 yards of fishing line you can use to make guitar strings. Each guitar string should be [ggfrac]1/3[/ggfrac] yard long. So, you can use 4 ÷ [ggfrac]1/3[/ggfrac] to find how many guitar strings you can make. You can draw 4 rectangles and divide each rectangle into 3 pieces (thirds). There are 12 thirds in all. You can make 12 guitar strings with the line. You notice that when you're finding the total number of thirds, the total is the same as the whole number TIMES the denominator of the unit fraction. Division and multiplication are opposite operations, like addition and subtraction. You can use multiplication to solve this division problem. To do that you KEEP 4 the same, CHANGE the sign to ×, and FLIP the [ggfrac]1/3[/ggfrac]. After you have done this, the new equation is 4 × [ggfrac]3/1[/ggfrac] , or 4 × 3. 4 × 3 = 12. That means that 4 ÷ [ggfrac]1/3[/ggfrac] is 12, so you can make 12 guitar strings. To find 6 ÷ [ggfrac]1/2[/ggfrac] , you can find 6 × [ggfrac]2/1[/ggfrac], or 6 × 2 = 12. Try this one yourself. For an art piece, you need one piece of ribbon that is [ggfrac]1/8[/ggfrac] inch long. You have 4 inches of ribbon. How many art pieces can you make with the amount of ribbon you have?

Pie Let’s say you have [ggfrac]1/2[/ggfrac] of a pie. You want to partition it into 3 equal pieces for 3 people. What fraction of the whole pie are the new pieces? Find [ggfrac]1/2[/ggfrac] ÷ 3. You can draw a circle to represent a whole pie and then draw a line to split the circle in half. Half of this circle represents the [ggfrac]1/2[/ggfrac] of a pie you start with. Now, partition that [ggfrac]1/2[/ggfrac] piece into 3 equal parts. When you do that, you can see that each smaller piece represents [ggfrac]1/6[/ggfrac] of the pie. That means that [ggfrac]1/2[/ggfrac] ÷ 3 = [ggfrac]1/6[/ggfrac]. Each of the 3 people get [ggfrac]1/6[/ggfrac] of a pie. Try this one yourself. You have [ggfrac]1/4[/ggfrac] of a piece of round cake. You want to partition that piece into 2 equal pieces. What fraction of the whole cake is each small piece?

Zen Garden Let’s say you are making desktop zen gardens. You have a ribbon that is [ggfrac]1/4[/ggfrac] yard long. You want to cut this piece of ribbon into 3 equal pieces, to use one of those pieces in each garden. You want to know how long the pieces are. To find out, you need to find [ggfrac]1/4[/ggfrac] ÷ 3. Use the KEEP CHANGE FLIP strategy to solve. You keep 14 the same, change the division sign to a multiplication sign, and flip 3, or [ggfrac]3/1[/ggfrac], to [ggfrac]1/3[/ggfrac]. [ggfrac]1/4[/ggfrac] × [ggfrac]1/3[/ggfrac]= [ggfrac]1/12[/ggfrac] . Each piece is [ggfrac]1/12[/ggfrac] yards long. Try this one yourself. You have [ggfrac]1/3[/ggfrac] foot of ribbon. You want to cut it into 2 equal pieces. What is the length of each smaller piece?